Calculations quiz
Calculations flashcardsCalculations practice – Molecular formulae
Refresh the page to get a different random selection of questions and answers.
1:15 know the structure of an atom in terms of the positions, relative masses and relative charges of sub-atomic particles
An atom consists of a central nucleus, composed of protons and neutrons.
This is surrounded by electrons, orbiting in shells (energy levels).
Atoms are neutral because the numbers of electrons and protons are equal.
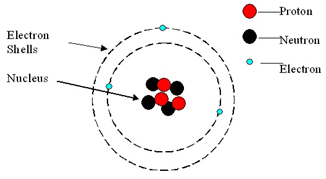
| Mass | Charge | |
|---|---|---|
| Proton | 1 | +1 |
| Neutron | 1 | 0 |
| Electron | negligible (1/1836) | -1 |
Atomic structure – Tyler de Witt video
This video explains the basics of atomic structure, telling you what is inside an atom:
1:16 know what is meant by the terms atomic number, mass number, isotopes and relative atomic mass (Aᵣ)
Atomic number: The number of protons in an atom.
Mass number: The number of protons and neutrons in an atom.
Isotopes: Atoms of the same element (same number of protons) but with a different number of neutrons.
Relative atomic mass (Ar): The average mass of an atom compared to 1/12th the mass of carbon-12.
1:17 be able to calculate the relative atomic mass of an element (Aᵣ) from isotopic abundances
75% of chlorine atoms are the type 35Cl (have a mass number of 35)
25% of chlorine atoms are of the type 37Cl (have a mass number of 37)
In order to calculate the relative atomic mass (Ar) of chlorine, the following steps are used:
- Multiply the mass of each isotope by its relative abundance
- Add those together
- Divide by the sum of the relative abundances (normally 100)
![\[ A_r = \frac{( (35 \times 75) + (37 \times 25) )}{100} \]](https://www.tutormyself.com/wp-content/ql-cache/quicklatex.com-0113f8af79de4c65c49213ff5f0f1314_l3.png "Rendered by QuickLaTeX.com")
![\[ A_r = 35.5 \]](https://www.tutormyself.com/wp-content/ql-cache/quicklatex.com-01950bf9b03b8605a6a2508f3e0a27ea_l3.png "Rendered by QuickLaTeX.com")
Example question:
A sample of bromine contained the two isotopes in the following proportions: bromine-79 = 50.7% and bromine-81 = 49.3%.
Calculate the relative atomic mass (Ar) of bromine.
![\[ A_r = \frac{( (79 \times 50.7) + (81 \times 49.3) )}{100} \]](https://www.tutormyself.com/wp-content/ql-cache/quicklatex.com-070b5d40d7a43c6544b0e3bdc25ecfcd_l3.png "Rendered by QuickLaTeX.com")
![\[ A_r = 79.99 \]](https://www.tutormyself.com/wp-content/ql-cache/quicklatex.com-9d467cc4bda5e7b370a0c2f489469759_l3.png "Rendered by QuickLaTeX.com")
Calculation of relative atomic mass – Tyler de Witt video
This excellent video from Tyler de Witt walks you through what isotopes are, and how the relative abundance of those isotopes can be used to calculate the relative atomic mass of an element.
1:19 understand how to deduce the electronic configurations of the first 20 elements from their positions in the Periodic Table
Electrons are found in a series of shells (or energy levels) around the nucleus of an atom.
Each energy level can only hold a certain number of electrons. Low energy levels are always filled up first.
Rules for working out the arrangement (configuration) of electrons:
Example – chlorine (Cl)
1) Use the periodic table to look up the atomic number. Chlorine’s atomic number (number of protons) is 17.
2) Remember the number of protons = number of electrons. Therefore chlorine has 17 electrons.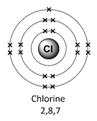
3) Arrange the electrons in levels (shells):
- 1st shell can hold a maximum of 2
- 2nd can hold a maximum of 8
- 3rd can also hold 8
Therefore the electron arrangement for chlorine (17 electrons in total) will be written as 2,8,7
4) Check to make sure that the electrons add up to the right number
The electron arrangement can also be draw in a diagram.
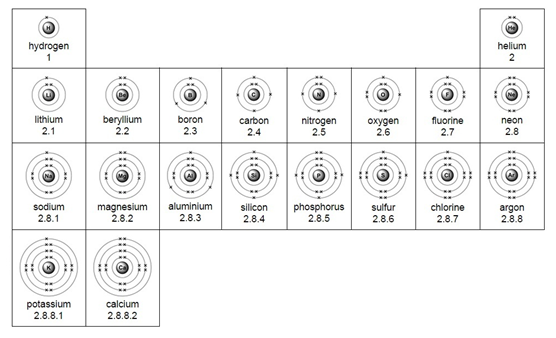
Electron arrangement for the first 20 elements:
1:26 calculate relative formula masses (including relative molecular masses) (Mᵣ) from relative atomic masses (Aᵣ)
Relative formula mass (Mr) is mass of a molecule or compound (on a scale compared to carbon-12).
It is calculated by adding up the relative atomic masses (Ar) of all the atoms present in the formula.
Example:
The relative formula mass (Mr) for water (H2O) is 18.
Water = H2O
Atoms present = (2 x H) + (1 x O)
Mr = (2 x 1) + (1 x 16) = 18
Calculation of relative formula mass – Tyler de Witt video
Here’s an excellent Tyler de Witt video explaining how to calculate the relative formula mass of compounds with:
- a simple formula
- a formula which includes brackets
- a formula which includes a dot (water of crystallisation)
Calculations involving mass (in grams), amount (in moles) and relative atomic mass – Tyler de Witt video
This video shows how to perform calculations involving mass (in grams), amount (in moles) and relative atomic mass:
1:31a understand how the formulae of simple compounds can be obtained experimentally, including metal oxides
Finding the formula of a metal oxide experimentally
The formulae of metal oxides can be found experimentally by reacting a metal with oxygen and recording the mass changes.
Example: When magnesium is burned in air, it reacts with oxygen (O2) to form magnesium oxide (MgO).
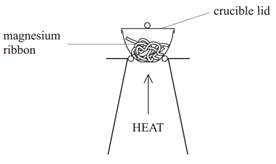
Method:
• Weigh a crucible and lid
• Place the magnesium ribbon in the crucible, replace the lid, and reweigh
• Calculate the mass of magnesium
(mass of crucible + lid + Magnesium – mass of crucible + lid)
• Heat the crucible with lid on until the magnesium burns
(lid prevents magnesium oxide escaping therefore ensuring accurate results)
• Lift the lid from time to time (this allows air to enter)
• Stop heating when there is no sign of further reaction
(this ensures all Mg has reacted)
• Allow to cool and reweigh
• Repeat the heating , cooling and reweigh until two consecutive masses are the same
(this ensures all Mg has reacted and therefore the results will be accurate)
• Calculate the mass of magnesium oxide formed (mass of crucible + lid + Magnesium oxide – mass of crucible + lid)
1:32 know what is meant by the terms empirical formula and molecular formula
The empirical formula shows the simplest whole-number ratio between atoms/ions in a compound.
The molecular formula shows the actual number of atoms of each type of element in a molecule.
For example, for ethane:
Molecular formula = C2H6
Empirical formula = CH3
Here are some more examples:
| Name | Molecular formula | Empirical Formula |
|---|---|---|
| pentene | C5H10 | CH2 |
| butene | C4H8 | CH2 |
| glucose | C6H12O6 | CH2O |
| hydrogen peroxide | H2O2 | HO |
| propane | C3H8 | C3H8 |
Notice from the table that several different molecules can have the same empirical formula, which means that it is not possible to deduce the molecular formula from the empirical formula without some additional information. Also notice that sometimes it is not possible to simplify a molecular formula into simpler whole-number ratio, in which case the empirical formula is equal to the molecular formula.
1:33 calculate empirical and molecular formulae from experimental data
Calculating the Empirical Formula
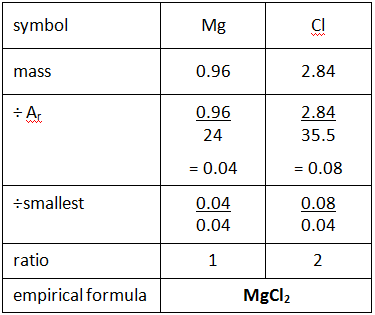Example: What is the empirical formula of magnesium chloride if 0.96 g of magnesium combines with 2.84 g of chlorine?
Step 1: Put the symbols for each element involved at the top of the page.
Step 2: Underneath, write down the masses of each element combining.
Step 3: Divide by their relative atomic mass (Ar).
Step 4: Divide all the numbers by the smallest of these numbers to give a whole number ratio.
Step 5: Use this to give the empirical formula.
(If your ratio is 1:1.5 then multiple each number by 2
If your ratio is 1:1.33 then x3. If your ratio is 1:1.25 x4)
Calculating the Molecular Formula
If you know the empirical formula and the relative formula mass you can work out the molecular formula of a compound.
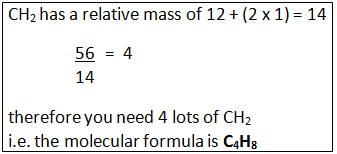
Example: A compound has the empirical formula CH2, and its relative formula mass is 56. Calculate the molecular formula.
Step 1: Calculate the relative mass of the empirical formula.
Step 2: Find out the number of times the relative mass of the empirical formula goes into the Mr of the compound.
Step 3: This tells how many times bigger the molecule formula is compared to the empirical formula.
Empirical formula calculations involving water of crystallisation
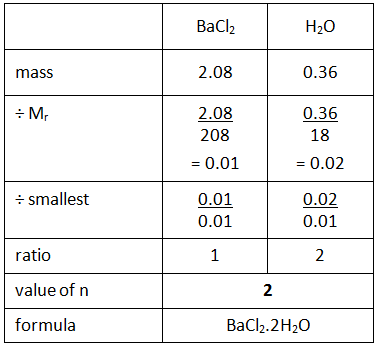
When you heat a salt that contains water of crystallisation, the water is driven off leaving the anhydrous (without water) salt behind. For example, barium chloride crystals contain water of crystallisation, and therefore would have the formula BaCl2.nH2O where the symbol ‘n’ indicates the number of molecules of water of crystallisation. This value can be calculated using the following method.
Example: If you heated hydrated barium chloride (BaCl2.nH2O) in a crucible you might end up with the following results.
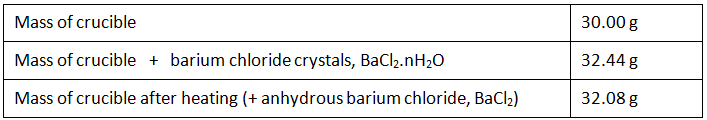
Step 1: Calculate the mass of the anhydrous barium chloride (BaCl2) and the water (H2O) driven off.
Step 2: Use the empirical formula method to find the value of n in the formula.
Empirical formulae and Molecular formulae – Tyler de Witt video
In this video, the lovely Tyler de Witt explains Empirical Formulae and Molecular formulae.
(Please excuse the horrid error right at the start where the video shows a diagram of prop-2-ene, but it is labelled ‘ethene’ by mistake.)
This second video then explains how, if given an empirical formula and a molecular mass, you can calculate the molecular formula:
1:36 practical: know how to determine the formula of a metal oxide by combustion (e.g. magnesium oxide) or by reduction (e.g. copper(II) oxide)
To determine the formula of a metal oxide by combustion.
Example: When magnesium is burned in air, it reacts with oxygen (O2) to form magnesium oxide (MgO).
2Mg + O2 –> 2MgO
Method:
- Weigh a crucible and lid
- Place the magnesium ribbon in the crucible, replace the lid, and reweigh
- Calculate the mass of magnesium
- Heat the crucible with lid on until the magnesium burns (lid prevents magnesium oxide escaping therefore ensuring accurate results)
- Lift the lid from time to time (this allows air to enter)
- Stop heating when there is no sign of further reaction
- Allow to cool and reweigh
- Repeat the heating, cooling and reweigh until two consecutive masses are the same (this ensures all Mg has reacted and therefore the results will be accurate)
- Calculate the mass of magnesium oxide formed (mass after heating – mass of crucible)
It is then possible to use this data to calculate the empirical formula of the metal oxide.
To determine the formula of a metal oxide by reduction
Example: When copper (II) oxide is heated in a stream of methane, the oxygen is removed from the copper (II) oxide, producing copper, carbon dioxide and water:
4CuO + CH4 –> 4Cu + CO2 + 2H2O
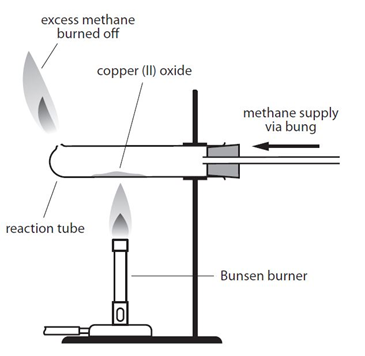
By comparing the mass of copper produced with the mass of copper oxide used it is possible to determine the formula of the copper oxide.
As an alternative, hydrogen gas could be used instead of methane:
CuO + H2 –> Cu + H2O
It is then possible to use this data to calculate the empirical formula of the metal oxide.
There is an important safety point to note with both versions of this experiment. Both methane and hydrogen are explosive if ignited with oxygen. It is important that a good supply of the the gas is allowed to fill the tube before the gas it lit. This flushes out any oxygen from the tube, so the gas will only burn when it exits the tube and comes into contact with oxygen in the air.